Clock And Calendar Test -

Clock And Calendar Test - Short-cut Tricks And Examples

The clock has 12 hours numbered from 1 to 12. Also, the clock is divided into 60 equal minute divisions. Therefore, each hour number is separated by five minute divisions.

If a watch or a clock indicates 8.15, when the correct time is 8, it is said to be 15 minutes too fast. On the other hand, if it indicates 7.45, when the correct time is 8, it is said to be 15 minutes too slow.

Minute Spaces

The face or dial of watch is a circle whose circumference is divided into 60 equal parts, called minute spaces.

Hour Hand and Minute Hand

A clock has two hands, the smaller one is called the hour hand or short hand while the larger one is called minute hand or long hand.

Relative position of the hands

The position of the M.H. relative to the H.H. is said to be the same, whenever the M.H. is separated from the H.H. by the same number of minute divisions and is on same side (clockwise or anticlockwise) of the H.H. Any relative position of the hands of a clock is repeated 11 times in every 12 hours.

1. When both hands are 15 minute spaces apart, they are at right angle.
2. When they are 30 minute spaces apart, they point in opposite directions.
3. The hands are in the same straight line when they are coincident or opposite to each other.

Incorrect Clock

If a clock indicates 6 : 10, when the correct time is 6 : 00, it is said to be 10 minute to fast and if it indicates 5 : 50 when the correct time is 6 : 00, it is said to be 10 minute slow.

If both hands coincide at an interval x minutes and 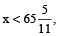 then total time gainedand clock is said to be ‘fast’.

If both hands coincide at an interval x minutes and then total time lost and clock is said to be ‘slow’.

Points to Remember

One minute division == 6° apart. i.e. In one minute, the minute hand moves 6°.

One hour division = 6° × 5 = 30° apart. i.e. In one hour, the hour hand moves 30° apart.

Also, in one minute, the hour hand moves = 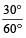= apart.

Since, in one minute, minute hand moves 6° and hour hand moves , therefore, in one minute, the minute hand gains 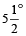 more than hour hand.

In one hour, the minute hand gains 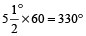over the hour hand. i.e. the minute hand gains 55 minutes divisions over the hour hand.

In every hour, both the hand coincide once.

In a day, the hands are coinciding 22 times.

In every 12 hours, the hands of clock coincide 11 times.

In every 12 hours, the hands of clock are in opposite direction 11 times.

In every 12 hours, the hands of clock are at right angles 22 times.

In every hour, the two hands are at right angles 2 times.

In every hour, the two hands are in opposite direction once.

In a day, the two hands are at right angles 44 times.

If both the hands coincide, then they will again coincide after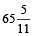minutes. i.e. in correct clock, both hand coincide at an interval of  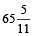minutes.

If the two hands coincide in time less than 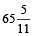 minutes, then clock is too fast and if the two hands coincides in time more than  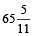minutes, then the clock is too slow.

Solved Examples

Question 1. Find the angle between the minute hand and hour hand of a clock when the time is 7.20

Solution: Angle traced by hour hand in 12 hours = 360 Degrees

Angle traced by it in 7 hrs 20 min i.e 22/3 hrs

= (360/12) × (22/3)= 2200

Angle traced by minute hand in 60 min = 3600

Angle traced by it in 20 minutes = (360/60) × 20 = 1200

Required angle = (2200 – 1200) = 1000

Question 2. At what time between 2 and 3 o’ clock will the hands of a clock together ?

Solution: At 2 o’ clock, the hour hand is at 2 and minute hand at 12, i.e., they are 10 minute spaces apart.

To be together , the minute hand must gain 10 minutes over the hour hand.

Now, 55 minutes are gaines by it in 60 minutes

10 minutes will be gained in {(60/55) × 10} min =10 10/11 min

The hands will coincide at 10 10/11 min past 2

Question 3. At what time between 4 and 5 o’ clock will the hands of a clock be at right angle ?

Solution: At 4 o’ clock, the minute hand will be 20 min spaces behind the hour hand.

Now , when the two hands are at right angles, they are 15 min. spaces apart.

So, they are at right angles in following two cases

Case I: When minute hand is 15 minute spaces behind the hour hand

In this case min hand will have to gain (20 –15) = 5 minute spaces

55 min. spaces will be gained by it in (60 × 5)/55 min = 5 5/11 min

They are at right angles at 5 5/1 min past 4

Case II: When the minute hand is 15 minute spaces ahead of the hour hand

They are at right angles at 38 2/11 min past 4

Question 4. At what time between 4 and 5 will the hands of a watch

(i) coincide, and (ii) point in opposite directions.

Solution:

(i) At 4 O’ clock, the hands are 20 minutes apart. Clearly the minute hand must gain 20 minutes before two hands can be coincident.

But the minute-hand gains 55 minutes in 60 minutes.

Let minute hand will gain x minute in 20 minutes.

So,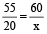 

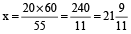⇒  min.

∴ The hands will be together at 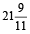 min past 4.

(ii) Hands will be opposite to each other when there is a space of 30 minutes between them. This will happen when the minute hand gains (20 + 30) = 50 minutes.

Now, the minute hand gains 50 min in 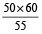 or  min.

∴  The hands are opposite to each other at 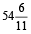 min past 4.

Question 5. What is the angle between the hour hand and minute hand when it was 5 : 05 pm.

Solution: 5.05 pm means hour hand was on 5 and minute hand was on 1, i.e. there will be 20 minutes gap.

∴  Angle = 20 × 6° = 120° [1 minute = 6°]

Question 6. My watch, which gains uniformly, is 2 min slow at noon on Sunday, and is 4 minutes 48 seconds fast at 2 pm on the following Sunday. When was it correct ?

Solution: From Sunday noon to the following Sunday at 2 pm

= 7 days 2 hours = 170 hours.

The watch gains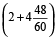=  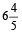minutes in 170 hours.

∴  The watch gains 2 minutes in 

Now, 50 hours = 2 days 2 hours.

2 days 2 hours from Sunday noon = 2 pm on Tuesday.

Question 7. The minute hand of a clock overtakes the hour hand at intervals of 65 minutes of the correct time. How much a day does the clock gain or lose?

Solution: In a correct clock, the minute hand gains 55 min. spaces over the hour hand in 60 minutes.

To be together again, the minute hand must gain 60 minutes over the hour hand.

55 min. are gained in 

But, they are together after 65 min.

∴  Gain in 65 min. 

Gain in 24 hours 

∴  The clock gains  minutes in 24 hours.

Question 8. A man who went out between 5 or 6 and returned between 6 and 7 found that the hands of the watch had exactly changed place. When did he go out?

Solution: Between 5 and 6 to 6 and 7, hands will change place after crossing each other one time. i.e., they together will make 1 + 1 = 2 complete revolutions.

H.H. will move through

 or  minute divisions.

Between 5 and 6 → minute divisions.

At 5, minute hand is 25 minute divisions behind the hour-hand.

Hence it will have to gain  minute divisions on the hour-hand

 minute divisions on the hour hand.

The minute hand gains minute divisions

 minutes  minutes

∴ The required time of departure is min past 5.

Calendar

The Julian calendar is a reform of the Roman calendar introduced by Julius Caesar in 46 BC (708 AUC). It took effect the following year, 45 BC (709 AUC), and continued to be used as the civil calendar in some countries into the 20th century. The calendar has a regular year of 365 days divided into 12 months, as listed in Table of months. A leap day is added to February every four years. The Julian year is, therefore, on average 365.25 days long.

The calendar year was intended to approximate the tropical (solar) year. Although Greek astronomers had known, at least since Hipparchus, that the tropical year was a few minutes shorter than 365.25 days, the calendar did not compensate for this difference. As a result, the calendar year gained about three days every four centuries compared to observed equinox times and the seasons. This discrepancy was corrected by the Gregorian reform, introduced in 1582.

The Julian calendar has been replaced by the Gregorian calendar in all countries which formerly used it as the civil calendar. Most Christian denominations have also replaced it with the Gregorian calendar as the basis for their liturgical calendars.

Points to Remember

Odd Days
We are supposed to find the day of the week on a given date. For this, we use the concept of ‘odd days’.In a given period, the number of days more than the complete weeks are called odd days.
Leap Year

• Every year divisible by 4 is a leap year, if it is not a century.
• Every 4th century is a leap year and no other century is a leap year.

Note: A leap year has 366 days.

Examples:

• Each of the years 1948, 2004, 1676 etc. is a leap year.
• Each of the years 400, 800, 1200, 1600, 2000 etc. is a leap year.
• None of the years 2001, 2002, 2003, 2005, 1800, 2100 is a leap year.

Ordinary Year

The year which is not a leap year is called an ordinary years. An ordinary year has 365 days.

Counting of Odd Days

• 1 ordinary year = 365 days = (52 weeks + 1 day.)
• 1 ordinary year has 1 odd day.
• 1 leap year = 366 days = (52 weeks + 2 days)
• 1 leap year has 2 odd days.
• 100 years = 76 ordinary years + 24 leap years = (76 × 1 + 24 × 2) odd days = 124 odd days. = (17 weeks + 5 days) = 5 odd days.
• Number of odd days in 100 years = 5.
• Number of odd days in 200 years = (5 × 2)→ 3 odd days.
• Number of odd days in 300 years = (5 × 3) → 1 odd day.
• Number of odd days in 400 years = (5 × 4 + 1)→ 0 odd day.
• Similarly, each one of 800 years, 1200 years, 1600 years, 2000 years etc. has 0 odd days.

Day of the Week Related to Odd Days:

No. of days:	0	1	2	3	4	5	6
Day:	Sun.	Mon.	Tues.	Wed.	Thurs.	Fri.	Sat.

Solved Examples

Question 1. What day of the week was 15th August 1949?

Solution: 15th August 1949 means

1948 complete years + first 7 months of the year 1949 + 15 days of August.

1600 years give no odd days.

300 years give 1 odd day.

48 years give {48 + 12} = 60 = 4 odd days.

[For ordinary years → 48 odd days and for leap year 1 more day (48 ÷ 4) = 12 odd days;

60 = 7 × 8 + 4]

From 1st January to 15th August 1949

Odd days : January – 3; February – 0; March – 3; April – 2; May – 3; June – 2; July – 3; August – 1

17 ⇒ 3 odd days.

∴ 15th August 1949 → 1 + 4 + 3 = 8 = 1 odd day.

This means that 15th Aug. fell on 1st day.

Therefore, the required day was Monday.

Question 2. How many times does the 29th day of the month occur in 400 consecutive years?

Solution: In 400 consecutive years, there are 97 leap years.

Hence, in 400 consecutive years, February has the 29th day 97 times and the remaining eleven months have the 29th day
400 × 11 = 4400 times

∴ The 29th day of the month occurs

(4400 + 97) or 4497 times.

Question 3. Today is 5th February. The day of the week is Tuesday. This is a leap year. What will be the day of the week on this date after 5 years?

Solution: This is a leap year. So, next 3 years will give one odd day each. then leap year gives 2 odd days and then again next year give 1 odd day.

Therefore (3 + 2 + 1) = 6 odd days will be there.

Hence the day of the week will be 6 odd days beyond Tuesday, i.e., it will be Monday.

Question 4. What day of the week was 20th June 1837?

Solution: 20th June 1837 means 1836 complete years

+ first 5 months of the year 1837 + 20 days of June.

1600 years give no odd days.

200 years give 3 odd days.

36 years give (36 + 9) or 3 odd days.

1836 years give 6 odd days.

From 1st January to 20th June there are 3 odd days.

Odd days : January — 3; February — 0; March — 3; April — 2; May — 3; June — 6

Total odd days =17 ⇒ 3 odd days.

Therefore, the total number of odd days = (6 + 3) or 2 odd days.

This means that the 20th of June fell on the 2nd day commencing from Monday.

Therefore, the required day was Tuesday.

Question 5. Prove that the calender for 1990 will same for 2001 also.

Solution: It is clear that the calender for 1990 will same for 2001 if first January of both the years is the same weekdays. For that the number of odd days between 31st December 1989 and 31st December 2000 must be zero. Odd days are as given below:

Year	1990	1991	1992	1993	1994	1995
Odd days	1	1	(L) 2	1	1	1
Year	1996	1997	1998	1999	2000
Odd days	(L) 2	1	1	1	(L) 2

Total number of odd days = 14 ⇒ 0 odd days.

 

 

Rankings and Time Sequence

Ranking And Time Sequence - Short-cut Tricks And Examples

Dear Reader, below are five simple types of ‘ranking and time sequence’ problems. You will find detailed solutions with each of the problems

As far as ranking problems are concerned, you have to remember one simple formula.

Total number of people = Rank of a person from START + Rank of person from the END – 1

Let we start with our tutorial. At the end of the tutorial, you will find a short practice test. Please do take the test so that you can be double sure that you have understood well.

Type I: Finding Rank From the Start (or the End)

In type 1, you will know the rank of a person from either the start (or the end). Using that data, you have to find the rank of that person from the end (or the start). Below example will help you.

Example Question 1: Reena is 10 ranks ahead of Priya in a class of 40. If Priya`s rank is 20th from the last, what is Reena`s rank from the start?
Answer: 31th

Solution:
Priya’s rank from the last is 20. Reena is 10 ranks ahead. Therefore, Reena’s rank from the last = Rank of Reena from the last = 20 – 10 = 10th
Now you have to apply the formula that you saw in the introduction.
Total number of students = Rank of Reena from the start + Rank of Reena from the end – 1
40 = Rank of Reena from the start + 10 – 1
Rank of Reena from the start = (40 – 10) +1
= 31th

Type II: Finding Total Number of People in a Sequence

In type 2, you will find ranks of a person from the start and the end. You have to find the total number of people. Let us see an example.

Example Question 2: Venkatesh ranks 8th from the top and 24th from the bottom in the class. How many students are there in total?
Answer: 31

Solution:
Total number of Students = Rank of Venkatesh from the top + Rank of Venkatesh from the bottom – 1
= [8 + 24] – 1= 31

Type III: Interchanging Positions

In this type, positions of the people in a sequence will be interchanged. You have to solve these problems after processing the data given.

Example Question 3: In a row of girls, Uma is 10th from the left and Meena is 20th from the right. If they interchange their positions, Uma becomes 15th from the left. How many girls are there in the row?
Answer: 34

Solution:
After interchange, rank of Uma from the left = 15
But, before interchange, Meena was 20th from the right. After interchange, Uma would have occupied the same position of Meena’s earlier spot. Therefore,
Present rank of Uma from the right = 20
Now you know the current ranks of Uma from left as well as right. Therefore,
Total number of girls = Uma’s rank from the left + Uma’s rank from the right – 1
= (15+20)-1
=34.

Type IV: Intervention in a Frequent Event

Though the heading of this type looks complicated, this is one of the easiest. There will be intervention at certain time of a frequent event. Based on that time data you have to solve the problem logically.

Let us see an example.

Example Question 4: A bus leaves to Chennai from Bangalore every 30 minutes. A passenger inquired about the next bus to Bangalore, and he was informed that the bus left 15 minutes before, and the next bus will be at 5.00 pm. Find at what time the passenger had enquired?
Answer: 4.45 pm

Solution:
For every 30 minutes, there is a bus. The next bus will be at 5.00 pm, so the last bus must have left at 4.30 pm.
The informer said that the bus had left 15 minutes before his inquiry. So the time of inquiry is 4.30 + 0.15 = 4.45 pm.

Type V: Day of Week Based on Frequency

This type is very easy just like the previous one. You can answer this with little effort. (You may not expect questions simple in your exam. However, this will be a first step in understanding more difficult questions.)

Example Question 5: Gita went to the temple five days ago. If she goes to the temple every Friday, then what day of the week is today?
Answer: Wednesday

Solution:
She went to temple five days ago i.e., Friday.
Five days from Friday is Wednesday.

Venn Diagram Test

Venn Diagram Test - Short-cut Tricks And Examples

Type 1: Matching the Relationship

You will find a set of things in the question. You have to find the best diagram that fits the connection between the things. Below is an example to help you.

Example Question 1: Which of the following diagram indicates the best relation between Pen, Nib and Pencil?

Answer: a
Reason:
The relation between pen, nib and pencil.
Nib is a component or part of pen and pencil is different from both.
Among the options, option a represents one circle inside the other i.e. nib is the part of pen and another circle individually which represent pencil that does not have relation with other two.

Type 2: Numbers (Data) Inside Diagram

In this type, you will find numbers (data) in Venn diagrams. You have to find the answers based on the data.

Below is an example question.

Example Question 2: In the below diagram, rectangle represents Dancer, triangle represents Singer and circle represents Dramatist.

1. How many dancers are also singers?
a) 7 b) 5 c) 8 d) 2
Answer: c) 8
Reason:
Rectangle represents dancers. Triangle represents singers. To find the dancers who are also singers, see the overlap area between rectangle and triangle.
The overlap contains 6 and 2. Therefore, the answer is 6+2 = 8.

2. How many dramatist are both dancers and singers?
a) 6 b) 5 c) 8 d) 2
Answer: a) 6

3. how many singers are neither dancers nor dramatist?
a) 6 b) 4 c) 8 d) 2
Answer: a) 8
Reason:
You have to find the area that represents only singers and neither dancer nor dramatists. Therefore, you observe the part in triangle that is not a part of rectangle or circle.
The part of triangle separate from rectangle and circle contains 8.
Therefore, 8 is the answer.

 

 

Seating Arrangement -

Seating Arrangement - Short-cut Tricks And Examples

In such problems a group of people, objects, etc, may have to be is arranged in a row or in a circle or any other way. Let us see the example given below:

Example: Just read the following informations carefully to answer the questions given below it:

Five friends P, Q, R, S, and T are sitting on a bench: (i) P is sitting next to Q. (ii) R is sitting next to S. (iii) S is not sitting with T. (iv) T is on the last end of the bench. (v) R is on the 2nd position from the right. (vi) P is on the right of Q and T. (vii) P and R are sitting together.

1. Where is P sitting?

1. Between S and R
2. Between S and R
3. Between T and S
4. Between S and T
5. Between Q and R

2. Who is sitting in the centre?

1. P
2. Q
3. R
4. S
5. T

3. R is sitting between……………

1. Q and S
2. P and T
3. S and T
4. P and S
5. P and Q

4. What is the position of S?

1. Extreme left
2. Extreme right
3. Third from left
4. Second from left
5. None of these

5. What is the position of Q?

1. 2nd from right
2. Centre
3. Extreme left
4. 2nd from left
5. None of these

Now, point to be noted that in arrangement problems the actual information can be classified into 2 categories:

1. Definite information: A definite information is one when the place of object/man is definitely mentioned.
2. Comparative information: In such information the place of object/man is not mentioned definitely but only a comparative position is given. In other words the positions of objects/men are given in comparison to another objects/men.

Now, to solve the problem go as per the following steps:

• Step I: Sketch a diagram of empty places
• Step II: Fill up as many empty places as possible using all the definite informations.
• Step III: With the help of comparative information consider all possibilities and select the possibilities which does not violate any condition.

Now, we can solve the given example:

Here 4th and 5th sentences constitute definite information:

Comparative informations are: 1st, 2nd, 6th and 7th sentences while 3rd is a negative information.

Now, start with definite information, sketch the following arrangement:

T __ __ R __

Now, this is the time to look for the comparative informations that tell about T and R. Such informations are 2nd, 6th and 7th sentences. Take the 7th and the 1st sentence. If P and R are together and also Q and P are together, then P must be between Q and R. Now the arrangement take the form as:

T Q P R ____

By the virtue of the 2nd sentence:

T Q P R S

Now, look at the given questions and check that you get the following answer:

1. (5)

2. (1)

3. (4)

4. (2)

5. (4)

Solved Examples

Directions: Study the following information carefully and answer the question given below:

A, B, C, D, E, F, G and H are sitting around a circle facing at the centre. D is second to the left of F and third to the right of H. A is second to the right of F and an immediate neighbour of H. C is second to the right of B and F is third to the right of B. G is not an immediate neighbour of F.

Question 1. How many of them, are there between H and C?

1. Two
2. Three
3. Two or Three
4. Data inadequate
5. None of these

Question 2. Who is to the immediate left of A?

1. H
2. E
3. G
4. Data inadequate
5. None of these

Question 3. In which of the following pairs is the first person sitting to the immediate left of the second person?

1. CD
2. BG
3. HA
4. FC
5. None of these

Question 4. Who is fourth to the right of B ?

1. E
2. C
3. A
4. Data inadequate
5. None of these

Question 5. What is E’s position with respect to G?

1. Second to the right
2. Third to the left
3. Third to the right
4. Second to the left
5. None of these

---

Solution (Q. 1-5): Seating arrangement is as follows:

Answers

1. (2)

2. (2)

3. (4)

4. (1)

5. (2)

---

Directions: On the basis of the information given below, select the correct alternative as answer for the questions which follow the information.

(i) A, B, C, D, E, F and G are sitting on a wall facing east. (ii) C is just right of D. (iii) B is on end point and E is his neighbour. (iv) G is sitting between E and F. (v) D is third from south end.

Question 6.Which of the following is a pair of parsons who is on end points?

1. AE
2. AB
3. FB
4. CB

Question 7. Which of the following information is not necessary to determine the position of A?

1. i
2. ii
3. iii
4. All informations are necessary

Question 8. D is sitting between which of the following pairs?

1. CE
2. AC
3. CF
4. AF

Question 9. C want his seat as third from north, he will have to exchange the seat from which person?

1. G
2. F
3. E
4. Cannot be determined

Question 10. Who is seated right side of E ?

1. F
2. D
3. C
4. G

---

Solutions (6-10): Given information diagrammatically can be shown as follows:

6. (2): A and B is a pair of persons who is at the end points.

7. (4): All informations are necessary

8. (3): D is sitted between C and F.

9. (4): He will have to exchange his seat from G.

10. (5): G is seated right side of E.

Analytical Puzzle Test

Analytical Puzzle Test - Short-cut Tricks And Examples

Type 1: Classification Type of Puzzles

In this type, you have to form a table and plot the information given in the question. Based on your table, you can quickly answer all the sub-questions. (Usually, these types of questions will have more than one sub-questions just like data interpretation problems.)

Below is your example question. This problem will help you in understanding better.

Example Question 1: Study the following information carefully and answer the questions that are given below:
Mahesh and Siva are good in French and English
Anu and Baskar are good in English and Japanese
Anu, Kishore and Raju are good in Japanese and Hindi
Raju and Latha are good in Japanese and Chinese
Kishore and Siva are good in Hindi and French

i. Who is good in Chinese, Japanese and Hindi?
a) Anu b) Kishore c) Raju d) Siva
Answer: c) Raju

Reason:
If you create a table based on the question information, you will get the one below.

Name	French	English	Japanese	Chinese	Hindi
Mahesh	✓	✓
Siva	✓	✓			✓
Anu		✓	✓		✓
Baskar		✓	✓
Kishore	✓		✓		✓
Raju			✓	✓	✓
Latha			✓	✓

From the table, we can say Raju knows Japanese, Hindi and Chinese.

For the below sub-questions, you do not have to solve from the start. You can answer just by looking at the table you already created.

ii. Which pair is good in French and Hindi?
a) Siva and Latha b) Kishore and Baskar
c) Siva and Raju d) Siva and Kishore
Answer: d) Siva and Kishore

iii. Who is good in French, Hindi and not in English?
a) Mahesh b) Anu c) Raju d) Kishore
Answer: d) Kishore

iv. Who is not good in French, Chinese and Hindi?
a) Baskar b) Mahesh c) Raju d) Siva
Answer: a) Baskar

v. Which of the following pair is good is in French and Japanese?
a) Mahesh and Baskar b) Kishore and Anu
c) Raju and Siva d) None of these
Answer: d) None of these

Reason:
Kishore is the only person who is good in both French and Japanese. In the question, they have asked to find a pair. So the answer is none of these.

Type II: Comparison Type Questions

In type 2, you will find a comparison between two or more people. For example, the comparison could be in the order of strength, height, age, etc. You have to apply the data to get the correct order. Once you have obtained the right order, you can answer all the following questions, quite quickly.

Here is your example.

Example Question 2: Read the following information and answer the questions given below:
Among five friends, Ravi is stronger than Mohit but weaker than Rohit.
Mani is the strongest.
Prem is stronger than Mohit and weaker than Ravi.

i. Who is the weakest person?
a) Mani b) Prem c) Rohit d) Mohit
Answer: d) Mohit

Reason:
From statement 1, Rohit > Ravi > Mohit
From Statement 2, Mani > Rohit > Ravi > Mohit
From Statement 3, Ravi > Prem > Mohit
Hence, the final arrangement is
Mani > Rohit > Ravi > Prem > Mohit

ii. Who is the second strongest person?
a) Ravi b) Mohit c) Rohit d) Mani
Answer: c) Rohit

Type III: Sequential Order of Events

In type 3, you will be ordering events in sequence. You have read the conditions carefully, work out the possibilities and arrive at a logical conclusion.

Now let us go to the example questions.

Example Question 3: Study the following information carefully and answer the questions that follow:

In an inter-school event, competitions for Singing, Dancing, Verse Writing, Fancy Dress, Quiz and Debate have to be conducted for 5 days.
(a) Singing should not be conducted on Thursday.
(b) Verse Writing should be held immediately after Debate.
(c) There should be a gap of two days between Quiz and Fancy Dress.
(d) Dancing should be conducted on Tuesday and should not be followed by Fancy dress.
(e) Singing should not be followed by fancy dress.

i. Which program is to be conducted on Wednesday?
a) Singing b) Verse Writing c) Quiz d) Debate
Answer: c) Quiz

Reason:
From the statements, it is clear that Dancing is conducted on Tuesday.

Two days gap should be there between Quiz and Fancy dress. For this condition, two possibilities are there. One is Monday and Thursday and the other is Wednesday and Saturday.

Verse-writing should have be followed by Debate. Here also two possibilities. One is Friday and Saturday and the other is Thursday and Friday.

Singing may be on either Monday or Wednesday.

There is another condition that is, Singing should not follow Fancy dress. If we take Monday and Thursday for Quiz and Fancy Dress, Singing will be on Wednesday, and it will be followed by Fancy dress. Hence, our condition will not be satisfied.

So, we go for the other possibility and is given in below table.

Competition	Day
Singing	Monday
Verse Writing	Friday
Debate	Thursday
Quiz	Wednesday
Fancy Dress	Saturday
Dancing	Tuesday

Now, you have arrived at the order of the events. Therefore, you can answer any number of sub-questions quickly after that. Below are few sub -questions to help you understand better.

ii. Which Competition is conducted on Friday?
a) Debate b) Dancing c) Quiz d) Verse Writing
Answer: d) Verse Writing

iii. In which day fancy dress competition is conducted?
a) Monday b) Saturday c) Tuesday d) Wednesday
Answer: b) Saturday

iv. After which competition does Quiz is to be conducted?
a) Fancy dress b) Dancing c) Debate d) Singing
Answer: c) Debate

v. Which competition is to be conducted first?
a) Dancing b) Verse writing c) Debate d) Singing
Answer: d) Singing

Mathematical Operation

Mathematical Operations - Short-cut Tricks And Examples

In such type of questions some relationships are shown with the help of certain symbols/notations and/ or mathematical signs. Each symbol or sign is defined clearly in the question statement itself. In other words, each symbol or sign is accorded two values –one real value and another assigned value of each symbol or sign and then solve the questions accordingly.

For example, Suppose the triangle (∆) means addition.

We know that triangle is a plane figure but here it has been assigned the value of addition (+).

Thus, 3 ∆ 5 ⇒ 3 + 5 = 8

In this way, two work out such questions substitute the assigned/ implied meanings of the symbol or sign and proceed accordingly.

How to Solve the questions

To solve this type of questions, substitute the real signs in the given expression and then solve the expression according to the BODMAS rule.
Order of Operations – BODMAS

1. 1st. B – Brackets, do all the maths contained in brackets first
2. 2nd. O – Orders, square roots, powers and anything else not listed
3. 3rd. D – Division, do your divisions now
4. 4th. M – Multiplication
5. 5th. A – Addition
6. 6th. S – Subtraction

Example: If + means ÷, × means –, ÷ means × and – means +, then, 8 + 6 × 4 ÷ 3 – 4 = ?

1. – 12
2. – 20/3
3. 12
4. 20/3

Solution. (3): Using the given symbols, we have:

Given expression: = 8 ÷ 6 – 4 × 3 + 4 = 4/3 – 4 × 3 + 4

= 4/3 – 12 + 4 = -20/3.

Type 1: Value of the Given Expression

Example 1: If ‘÷’ means ‘+’, ‘–’ means ‘÷’, ‘×’ means ‘–’ and ‘+’ means ‘×’ then, 62 ÷ 8 – 4 × 12 + 4 = ?

1. 16
2. 26
3. 1/16
4. 6

Solution: (1) Given expression, 62 ÷ 8 – 4 × 12 + 4 = ?

According to question, after replacement of mathematical sign

62 + 8 ÷ 4 – 12 × 4 = ?

= 64 – 48 = 16

Hence, ? ⇒ 16

Type 2: Identification of Correct Equation

Example 2: If ‘–’ means ‘+’, ‘+’ means ‘–’, ‘×’ means ‘÷’ and ‘÷’ means ‘×’; then which of the given equations is correct?

1. 30 + 5 – 4 ÷ 10 × 5 = 58
2. 30 + 5 ÷ 4 – 10 × 5 = 22
3. 30 – 5 + 4 ÷ 10 × 5 = 62
4. 30 × 5 – 4 ÷ 10 + 5 = 41

Solution. (4): From option 4:

30 × 5 – 4 ÷ 10 + 5 = 41

According to question, after replacement of mathematical sign

30 ÷ 5 + 4 × 10 – 5 = 41 = 6 + 40 – 5 = 41 = 46 – 5 = 41

Hence option (4) is correct.

Solved Examples

Example 1: If ‘M’ means ‘÷’, R means ‘+’,  T means ‘-’, and ‘K’ means ‘×’ then what will be the value of the following expression?

20 R 16 K 5 M 10 T8 = ?

1. 36
2. 20
3. 36.5
4. 12

Solution. (2): ? = 20 + 16 × 5 ÷ 10 – 8

or  ? = 20 + 16 ×5/10– 8

or  ? = 20 + 8 – 8 = 20

Example 2: Of the two subjects offered to a class in their final year, 32 students in all are studying Psychology while a total of 26 students are studying Sociology. If 16 students have opted to specialize in both, what is the strength of the class?

1. 74
2. 58
3. 42
4. Date inadequate

Solution. (3): Venn diagram of given information would be as follows.

Total strength of the class = 16 + 16 + 10 = 42

Example 3: How many numbers would remain if the numbers which are divisible by 2 and also those having ‘2’ as only one of the digits are dropped from numbers 1 to 30?

1. 14
2. 17
3. 15
4. 10

Solution. (4):

 

 

Ranking And Ordering

Ranking And Ordering - Short-cut Tricks And Examples

The position of a thing/person etc. in a definite order is called as ‘Rank’.

In this type of test, relative position or rank of some person or object are given and candidates are required to find the rank or position of other person or object.

Type of Questions

Type 1. Rank of a person in a queue

Position of person from upward	= [Total no. of persons – position of person from down] + 1.
Position of person from downward	= [Total no. of persons – position of person from up] + 1.
Position of person from right	= [Total no. of persons – position of person from left] + 1.
Position of person from left	= [Total no. of persons – position of person from right] + 1.

Example 1: Anita ranks twelfth in a class of forty six. What will be her rank from the last?

1. 34th
2. 35th
3. 36th
4. 37th

Solution. (2): Rank of Anita from the last

= [Total students – her rank from first] + 1

= (46 – 12) + 1 = 35th.

Example 2: Ravi is 7 ranks ahead of Sumit in a class of 39. If Sumit’s rank is 17th from the last, what is Ravi’s rank from the start?

1. 14th
2. 15th
3. 16th
4. 17th

Solution. (c): Rank of Ravi from the last = 17 + 7 = 24th.

∴  Rank of Ravi from the start = (39 – 24) + 1 = 16th.

Type 2. Total number of person in a queue

Total no. of persons = [Position of person from upward/right + Position of person from downward/left] – 1.

Example 3: Rakesh ranks 7th from the top and 28th from the bottom in a class. How many students are there in the class?

1. 34
2. 35
3. 36
4. 37

Solution. (1): Total no. of students = [7 + 28] – 1 = 34.

Type 3. When two persons change their rank in a queue

If two persons are on a definite position from up and down (or left and right) and they interchange their ranks, then Total no. of persons in order = [present position of first person + previous position of second person] – 1

Example 4: In a row of girls, Shilpa is eighth from the left and Reena is seventeenth from the right. If they interchange their positions, Shilpa becomes fourteenth from the left. How many girls are there in the row?

1. 34
2. 35
3. 30
4. 37

Solution. (3): Total no. of girls

= [present position of Shilpa + previous position of Reena] – 1

= (14 + 17) – 1 = 30

Previous position of first person or present position of second person = Difference of two positions of second person + previous position of second person = Difference of two positions of first person + previous position of second person.

Example 5: In a row of children, Dipa is fifth from the left and Vijay is sixth from the right. When they interchange their places among themselves, Dipa becomes thirteenth from the left. Then, what will be Vijay’s position from the right ?

1. 4th
2. 14th
3. 8th
4. 12th

Solution. (b): Present position of Vijay

= Difference of two positions of Dipa + previous position of Vijay

= (13 – 5) + 6 = 14th

Solved Examples

Question 1. Aruna ranks twelfth in a class of fortysix. What will be her rank from the last?

Solution: Rank of Aruna from the last

= [Total student – her rank from first] + 1 = (46 – 12) + 1 = 35th.

Question 2. In a class of 35 students Kiran is placed 7th from the bottom whereas Sohan is placed 9th from the top. Mohan is placed exactly in between the two. What is Kiran’s position from Mohan ?

Solution: Position of Kiran from the top = [35 – 7] + 1 = 29 th

Position of Sohan from the top = 9th.

Difference of their positions = 29 – 9 = 20

∴ Mohan’s position from top = 9 + 10 = 19th

Hence, Kiran’s position from Mohan = 29 – 19 = 10th

Question 3. Sonal ranks 7th from the top and 28th from the bottom in a class. How many students are there in the class?

Solution: Total no. of students = [7 + 28] – 1 = 34.

Question 4. There are thirty five students in a class. Suman ranks third among the girls in the class. Amit ranks 5th among the boys in the class. Suman is one rank below Amit in the class. No two students hold the same rank in the class. What is Amit’s rank in the class?

Solution: Suman is one rank below Amit in the class. Hence two girls and four boys are ahead of Amit. Hence Amit’s rank in the class is 7th.

Question 5. Manish ranked sixteenth from the top and twenty-ninth from the bottom among those who passed an examination. Six boys did not participate in the competition and five failed in it. How many boys were there in the class?

Solution: Total no. of students = [(16 + 29) – 1] + 6 + 5 = 55.

Question 6. In a sequence of children, Kashish is fifth from the left and Mona is sixth from the right. When they interchange their places among themselves, Kashish becomes thirteenth from the left. Then, what will be Mona’s position from the right?

Solution: Second position of Mona = Difference of two positions of Kashish + First position of Mona = (13 – 5) + 6 = 14th

Question 7. Mohan is older than Prabir, Suresh is younger than Prabir. Mihir is older than Suresh but younger than Prabir. Who among the four is the youngest?

Solution:

• Mohan > Prabir > Suresh
• Prabir > Mihir > Suresh

Hence, Mohan > Prabir > Mihir > Suresh

Alphabet Reasoning

Alphabet Reasoning 

Type I: Word Formation From Letters of Another Word

In this type, you have to form words from specific letters of a given word. Next, you need to answer questions based on the new word/words formed. Below is an example.

Example Question 1: If it is possible to make a meaningful word with the fourth, the seventh, the eighth and the twelfth letters of the word “QUANTITATIVE”, which of the following will be the second letter of that word from the left end? If no such word can be made give ‘X’ as the answer and, if more than one such word can be made, give ‘M’ as the answer.
a) E b) M c) A d) X

Solution:
The given word is,

Q	U	A	N	T	I	T	A	T	I	V	E
1	2	3	4	5	6	7	8	9	10	11	12

Fourth letter in the word is – N
Seventh letter is – T
Eighth letter is – A
And twelfth letter is – E
The meaningful word you can form using the above letters is NEAT.
(Here, we can make only one word). So, the second letter from the left end is E.
Hence, the answer is Option a) E.

Type II: Letter Series

In this type, you have to find missing letters in a series. Let us see an example.

Example Question 2: What will come in the place of (?) in the following letter series?
EG, HJ, KM, NP, ?

Solution:
The letters in the series are formed by using the logic,
G is the second letter from E.
The second term (‘HJ’) starts with the successive letter of G, i.e., H.
Below diagram will help you to understand the logic behind the series better.

So the next letter in the series is QS. (Q is the next successive letter of P and S is the second letter from Q).

Type III: Identifying Letters That Match Given Conditions

In this type, you have to identify letters based on certain conditions given in the question. Here is an example.

Example Question 3: How many pairs of letters are there in the word “QUANTITATIVE” which have as many letters between them as in the English alphabet series?

Solution:
In the word, QUANTITATIVE you have to check for the pairs of letters that are in alphabet series.

In real alphabet series, the letters T and V have only one letter U between them.
In the word ‘QUANTITATIVE’ the letters T and V have only one letter I between them.
Therefore, TV is one such pair.

The other pair is AE. In the alphabet series, we have 3 letters between A and E., i.e., A, B, C, D and E.
Similarly, in the given word also, there are three letters between A and E.

Below diagram will help you to understand the solution better.

Hence, we have two such pair of letters (TV and AE).

Type IV: Arranging Words in Alphabetic Order

This type is the easiest of all. You have to arrange given words in alphabetical order and answer the corresponding questions.

Example Question 4: Arrange the following five words in alphabetical order as per dictionary then find which of the following word will come at the third place?
1) Music
2) Modern
3) Mobile
4) Manifest
5) Magic

Solution:
First, we have to arrange the words in dictionary (alphabetical) order.
First, check the first letter of the given words,

Words	Letters
Music	M
Modern	M
Mobile	M
Manifest	M
Magic	M

Here all the words start with the letter M. Therefore we have to move to the second letter to arrange in order.

Words	Letters
Music	U
Modern	O
Mobile	O
Manifest	A
Magic	A

Based on the second letter, the words can be arranged as,
Manifest
Magic
Modern
Mobile
Music
Here, the second letter of the words ‘Manifest’ and ‘Magic’ are same. Similarly, for ‘Modern’ and ‘Mobile’ the second letters are the same. In order, to find the proper arrangement we have to check with the third letter of these words.

Words	Letters
Manifest	N
Magic	G
Modern	D
Mobile	B

Now the arrangement is,
Magic
Manifest
Mobile
Modern
Music
The word that comes at the third place is ‘Mobile’.

Type V: Arrangement of Letters and Interpretation

In this type, you will find conditions based on which you have to arrange letters. Then you have to answer the questions based on the new arrangement. Below example will help you to understand clearly. (There are 3 sub questions to the below one.)

Example Question 5: Directions (i- iii) Read the following information carefully and answer the questions given below:
ABCDEFGHIJKLMNOPQRSTUVWXYZ

Write the first 10 letters of above alphabets in reverse order, followed by next 6 letters, followed by remaining 10 letters in reverse order.

(i). Which letter will be the thirteenth to the right of the third letter from right?
Solution to sub-question (i):
First, we have to arrange the letters based on the condition given.
Step 1 : Arrange first 10 letters in reverse order.
JIHGFEDCBA
Step 2: Next 6 letters should be followed as it is,
JIHGFEDCBAKLMNOP
Step 3: The remaining 10 letters should be reversed
JIHGFEDCBAKLMNOPZYXWVUTSRQ

Now, we have arranged the alphabets according to the question.
Next, we have to find the thirteenth letter right to the third letter from your right. You can find it using the diagram(i) shown below.

The required letter is K.

(ii). Four of the following five are alike in a certain way and form a group. Which is the one that does not belong to the group?
a) FDA b) LNZ c) PYV d) OZX e) WUR

Solution to sub question (ii):
In the arrangement we just created. (refer to diagram in sub-question (i))
Consider the first option ‘FDA. Here, D is the second letter from F and A is the third letter from D. The same logic is used to form all the terms except option d) OZX.
Below diagram will help you to understand the logic better.

(iii). How many letters are there in between letters F and O in the sequence?

Solution to sub-question (iii):
Based on our arrangement diagram (refer to the diagram in sub-question (i)), the letters in the sequence are,

F

E

D

C

B

A

K

L

M

N

O

The number of letters between F and O is 9.

Direction Sense

Direction Sense - Short-cut Tricks And Examples

Type I: Identify Direction (After Journey)

In this type, based on the conditions given, you have to construct a diagram. Based on your diagram, you have to identify the direction at final spot. Below example will help you to understand better.

Example Question 1: Rahul walks 5 km West, turns southeast and walks 8 km. Again, he turns towards northeast and walks 10 km and then turns to his right. In which direction from his starting point is he standing now?
a) East
b) West
c) Northeast
d) Southeast
Answer: c) Northeast

Reason:

If you apply the conditions given in the question, you will get the below diagram.

From the above diagram, you can say that Rahul is in Northeast direction from his starting point.

Type II: Identify Distance When Start & Finish Points Are in Straight Line

In this type, you will be asked to find the distance between start and finish points. The conditions will be such that the final point will be in straight line with the starting point. Below is an example for this type.

Example Question 2: Sita started from her home towards North and covered a distance of 200 m and then turned to her right and walked 150 m. Then she again turned to her right and walked 75 m and finally she again turned to her right and walked 150 m. How far is she from her home?
a) 125 m
b) 200 m
c) 175 m
d) 150 m
Answer: b) 125 m

Reason:
Based on the question, you will be able to draw the below diagram. As you can see, the starting point A is in straight line with the end point E.

As you know, the above diagram represents the total distance walked by Sita.
Now, we have to find the distance between Sita’s home and the place where she ends i.e., the distance of AE.
Here, AB = 200 m, CD = 75 m
Since CD and BE are parallel lines and BCDE is a rectangle, you can write BE = (CD =) 75 m.

Therefore, you can calculate AE as follows.
AE = AB – BE
= 200 – 75
=125 m.

Type III: Calculate Distance When Start & Finish Points Are NOT in Straight Line

This type is a slight extension of type 2. In this case, the start and finish points will not be in straight line. Below is an example.

Example Question 3: Kishore rides in his bicycle from his home 10 m towards north, then he turns right and travels 15 m, then turns left and travels 10 m and reaches his office. How far is the office from his home?
a) 100 m
b) 25 m
c) 75 m
d) 50 m
Answer: b) 25 m

Reason:

We have to find the distance between Kishore’s home and office.
From the diagram, the distance to find is AD.
From Pythagoras formula,
AD² = AE² + ED² ….. equation 1

But, from the above diagram, ED = EC + CD
ED = 10 + 10 (Because EC = AB = 10 and CD = 10)
= 20

Now you have to substitute the value of ED = 20, in equation 1.
AD² = 15² + 20²
AD² = 625
AD = 25 m

Type IV: Calculating Direction/Distance When Turns Are Given in Degrees

This type is similar to the above types with one exception. In this case, the turns will be given in degrees. Now let us see an example.

In such problems, whenever you see “clockwise”, you have to understand that the person is turning “right”. Similarly, an “anti-clockwise” turn denotes a “left” turn.

Example Question 4: Gita runs towards north 5 km and from there turned 90˚ anti-clockwise and runs 4 km. Then she takes 135˚ turn clockwise and ran 2 km. Find the direction of Gita with respect to the starting point.
a) South west Region
b) North west Region
c) South east Region
d) North east Region
Answer: d) North east Region

Reason:

You can easily draw the below diagram if you follow the question carefully.

Hence, the final direction is North East.